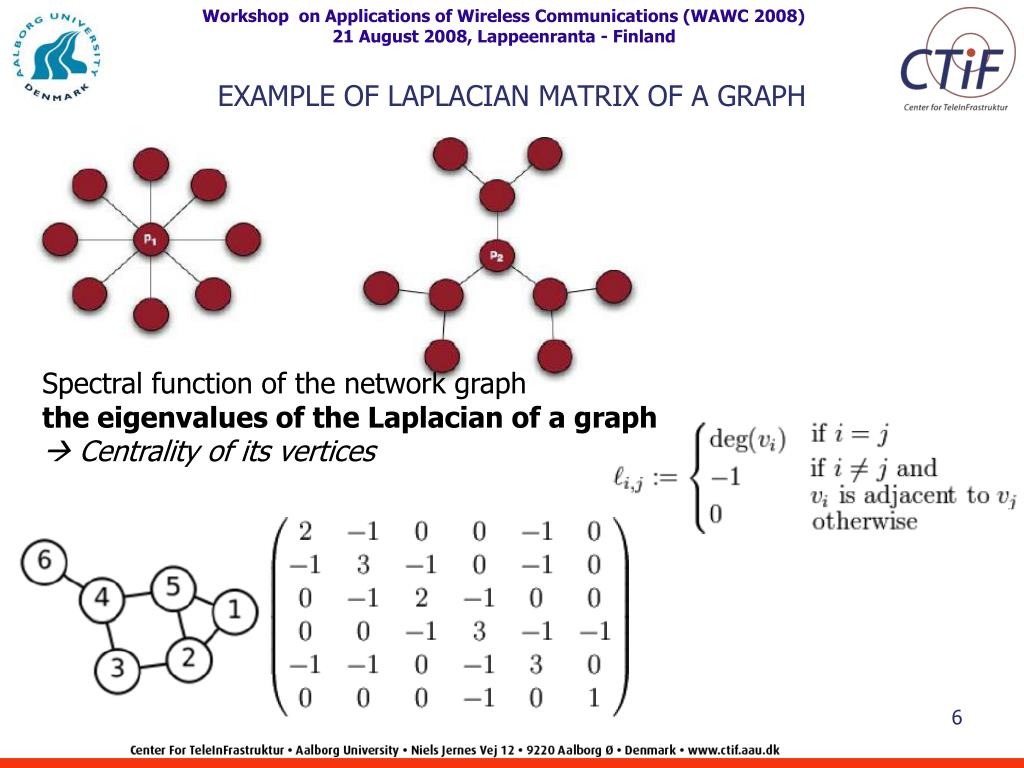
Network Graph Laplacian . A directed graph is a pair g = (v, e), where v = {v1,. It’s useful in studying random walks and. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. the graph laplacian is another important matrix representation of a network. Its laplacian matrix, l, can be defined in terms of the degree. graphs and graph laplacians. consider a graph, g = (v, e), having a finite number of v vertices and e edges.
from www.slideserve.com
graphs and graph laplacians. consider a graph, g = (v, e), having a finite number of v vertices and e edges. Its laplacian matrix, l, can be defined in terms of the degree. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. the graph laplacian is another important matrix representation of a network. A directed graph is a pair g = (v, e), where v = {v1,. It’s useful in studying random walks and.
PPT CONTROLLING EPIDEMICS IN WIRELESS NETWORKS PowerPoint
Network Graph Laplacian consider a graph, g = (v, e), having a finite number of v vertices and e edges. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. the graph laplacian is another important matrix representation of a network. consider a graph, g = (v, e), having a finite number of v vertices and e edges. Its laplacian matrix, l, can be defined in terms of the degree. graphs and graph laplacians. A directed graph is a pair g = (v, e), where v = {v1,. It’s useful in studying random walks and.
From royalsocietypublishing.org
Directed network Laplacians and random graph models Royal Society Network Graph Laplacian graphs and graph laplacians. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. consider a graph, g = (v, e), having a finite number of v vertices and e edges. It’s useful in studying random walks and. Its laplacian matrix, l, can be defined in terms of the degree. A directed graph. Network Graph Laplacian.
From www.researchgate.net
The steps of Laplacian eigenmaps algorithm. 13 Neighborhood graph Network Graph Laplacian It’s useful in studying random walks and. the graph laplacian is another important matrix representation of a network. A directed graph is a pair g = (v, e), where v = {v1,. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. graphs and graph laplacians. consider a graph, g = (v,. Network Graph Laplacian.
From www.semanticscholar.org
Figure 2 from Network Distance based on Laplacian Flows on Graphs Network Graph Laplacian graphs and graph laplacians. Its laplacian matrix, l, can be defined in terms of the degree. A directed graph is a pair g = (v, e), where v = {v1,. It’s useful in studying random walks and. the graph laplacian is another important matrix representation of a network. consider a graph, g = (v, e), having a. Network Graph Laplacian.
From deepai.org
Robust Graph Neural Networks using Weighted Graph Laplacian DeepAI Network Graph Laplacian Its laplacian matrix, l, can be defined in terms of the degree. It’s useful in studying random walks and. the graph laplacian is another important matrix representation of a network. graphs and graph laplacians. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. consider a graph, g = (v, e), having. Network Graph Laplacian.
From www.youtube.com
Graph Laplacian 1 (Unnormalized) Laplacian YouTube Network Graph Laplacian graphs and graph laplacians. the graph laplacian is another important matrix representation of a network. It’s useful in studying random walks and. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. consider a graph, g = (v, e), having a finite number of v vertices and e edges. A directed graph. Network Graph Laplacian.
From www.researchgate.net
Eigenvalue distribution of the Laplacian matrix of ErdősRényi random Network Graph Laplacian graphs and graph laplacians. consider a graph, g = (v, e), having a finite number of v vertices and e edges. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. Its laplacian matrix, l, can be defined in terms of the degree. the graph laplacian is another important matrix representation of. Network Graph Laplacian.
From www.researchgate.net
Communication graph G corresponding to Laplacian matrix W Download Network Graph Laplacian Its laplacian matrix, l, can be defined in terms of the degree. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. the graph laplacian is another important matrix representation of a network. graphs and graph laplacians. A directed graph is a pair g = (v, e), where v = {v1,. It’s useful. Network Graph Laplacian.
From deepai.org
sMGC A ComplexValued Graph Convolutional Network via Network Graph Laplacian the graph laplacian is another important matrix representation of a network. A directed graph is a pair g = (v, e), where v = {v1,. Its laplacian matrix, l, can be defined in terms of the degree. It’s useful in studying random walks and. the laplacian allows a natural link between discrete representations, such as graphs, and continuous.. Network Graph Laplacian.
From mbernste.github.io
The graph Laplacian Matthew N. Bernstein Network Graph Laplacian the graph laplacian is another important matrix representation of a network. Its laplacian matrix, l, can be defined in terms of the degree. It’s useful in studying random walks and. A directed graph is a pair g = (v, e), where v = {v1,. graphs and graph laplacians. consider a graph, g = (v, e), having a. Network Graph Laplacian.
From zhuanlan.zhihu.com
论文笔记 ICML 2022 pLaplacian Based Graph Neural Networks 知乎 Network Graph Laplacian It’s useful in studying random walks and. A directed graph is a pair g = (v, e), where v = {v1,. consider a graph, g = (v, e), having a finite number of v vertices and e edges. the graph laplacian is another important matrix representation of a network. Its laplacian matrix, l, can be defined in terms. Network Graph Laplacian.
From stats.stackexchange.com
conv neural network Difference between Symmetrically normalized Network Graph Laplacian consider a graph, g = (v, e), having a finite number of v vertices and e edges. A directed graph is a pair g = (v, e), where v = {v1,. the graph laplacian is another important matrix representation of a network. It’s useful in studying random walks and. Its laplacian matrix, l, can be defined in terms. Network Graph Laplacian.
From thenlp.space
Graph Neural Networks for beginnners — The NLP Space Network Graph Laplacian Its laplacian matrix, l, can be defined in terms of the degree. graphs and graph laplacians. the graph laplacian is another important matrix representation of a network. consider a graph, g = (v, e), having a finite number of v vertices and e edges. the laplacian allows a natural link between discrete representations, such as graphs,. Network Graph Laplacian.
From deepai.org
Network Distance Based on Laplacian Flows on Graphs DeepAI Network Graph Laplacian Its laplacian matrix, l, can be defined in terms of the degree. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. consider a graph, g = (v, e), having a finite number of v vertices and e edges. graphs and graph laplacians. A directed graph is a pair g = (v, e),. Network Graph Laplacian.
From www.researchgate.net
(PDF) Graph Laplacian and Neural Networks for Inverse Problems in Network Graph Laplacian A directed graph is a pair g = (v, e), where v = {v1,. consider a graph, g = (v, e), having a finite number of v vertices and e edges. Its laplacian matrix, l, can be defined in terms of the degree. graphs and graph laplacians. the graph laplacian is another important matrix representation of a. Network Graph Laplacian.
From math.stackexchange.com
matrices Network Theory Laplacian, Incidence Matrix, or what else Network Graph Laplacian the graph laplacian is another important matrix representation of a network. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. consider a graph, g = (v, e), having a finite number of v vertices and e edges. Its laplacian matrix, l, can be defined in terms of the degree. graphs and. Network Graph Laplacian.
From www.catalyzex.com
Advancing Graph Neural Networks with HLHGAT A HodgeLaplacian and Network Graph Laplacian consider a graph, g = (v, e), having a finite number of v vertices and e edges. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. Its laplacian matrix, l, can be defined in terms of the degree. It’s useful in studying random walks and. the graph laplacian is another important matrix. Network Graph Laplacian.
From guygilboa.net.technion.ac.il
Graph Laplacian for SemiSupervised Learning, accepted to SSVM2023 Network Graph Laplacian the laplacian allows a natural link between discrete representations, such as graphs, and continuous. graphs and graph laplacians. consider a graph, g = (v, e), having a finite number of v vertices and e edges. It’s useful in studying random walks and. Its laplacian matrix, l, can be defined in terms of the degree. the graph. Network Graph Laplacian.
From www.youtube.com
The Laplacian Matrices of Graphs Algorithms and Applications YouTube Network Graph Laplacian A directed graph is a pair g = (v, e), where v = {v1,. the laplacian allows a natural link between discrete representations, such as graphs, and continuous. It’s useful in studying random walks and. Its laplacian matrix, l, can be defined in terms of the degree. graphs and graph laplacians. consider a graph, g = (v,. Network Graph Laplacian.